Background independence
Background independence, also called universality, is the concept or assumption, fundamental to all physical sciences, that the nature of reality is consistent throughout all of space and time. More specifically, no observer can, under any circumstances, perform a measurement that yields a result logically inconsistent with a previous measurement, under a set of rules that are independent of where and when the observations are made.
This is not a suggestion that spacetime is uniform, merely that the fundamental rules governing the measurable characteristics of the physical universe are the same everywhere, at all times.
Contents |
Principle of universality
Prior to Albert Einstein using the principle in his Relativity, the idea had been considered by a number of theorists, such as David Hume, Charles Lyell, and James Hutton, under the name principle of universality. It postulates that the laws of nature discovered on Earth apply throughout the universe.
There are several variations and corollaries:
- A stronger uniformity principle is that the laws of event causation have remained constant throughout time (uniformitarianism) as well as applying everywhere in the 'modern' universe.
- A corollary in physics is the postulate that there has been no change in the fine-structure constant since the Big Bang.
- Another corollary of the principle of uniformity states that everything that is now possible in principle was ever the case in the past.
Diffeomorphism invariance and background independence
The argument involves only the very basics of general relativity (GR), as we will see below. More details and discussions can be found in Rovelli's book or the papers by Rovelli and Gaul[1] and by Smolin.[2]
It begins with an utterly trivial mathematical observation. Here is written the differential equation for the simple harmonic oscillator twice
- Eq(1)
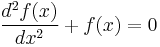
- Eq(2)
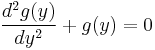
except in Eq(1) the independent variable is x and in Eq(2) the independent variable is  . Once we find out that a solution to Eq(1) is
. Once we find out that a solution to Eq(1) is  , we immediately know that
, we immediately know that 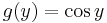 solves Eq(2). This observation combined with general covariance has profound implications for GR.
solves Eq(2). This observation combined with general covariance has profound implications for GR.
Assume pure gravity first. Say we have two coordinate systems,  -coordinates and
-coordinates and  -coordinates. General covariance demands the equations of motion have the same form in both coordinate systems, that is, we have exactly the same differential equation to solve in both coordinate systems, except in one the independent variable is
-coordinates. General covariance demands the equations of motion have the same form in both coordinate systems, that is, we have exactly the same differential equation to solve in both coordinate systems, except in one the independent variable is  and in the other it is
and in the other it is  . Once we find a metric function
. Once we find a metric function 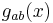 that solves the EOM in the
that solves the EOM in the  -coordinates we immediately know (by exactly the same reasoning as above!) that the same function written as a function of
-coordinates we immediately know (by exactly the same reasoning as above!) that the same function written as a function of  solves the EOM in the
solves the EOM in the  -coordinates. As both metric functions have the same functional form but belong to different coordinate systems, they impose different spacetime geometries. Thus we have generated a second distinct solution!
-coordinates. As both metric functions have the same functional form but belong to different coordinate systems, they impose different spacetime geometries. Thus we have generated a second distinct solution!
Now comes the problem. Say the two coordinate systems coincide at first, but at some point after 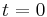 we allow them to differ. We then have two solutions, they both have the same initial conditions yet they impose different spacetime geometries. The conclusion is that GR does not determine the proper time between spacetime points! This argument (or rather a refinement of it) is known as Einstein's hole argument. It is straightforward to include matter - we have a larger set of differential equations but they still have the same form in all coordinates systems, so the same argument applies and again we obtain two solutions with the same initial conditions which impose different spacetime geometries. It is very important to note that we could not have generated these extra distinct solutions if spacetime were fixed and non-dynamic, and so the resolution to the hole argument, background independence, only comes about when we allow spacetime to be dynamic.
we allow them to differ. We then have two solutions, they both have the same initial conditions yet they impose different spacetime geometries. The conclusion is that GR does not determine the proper time between spacetime points! This argument (or rather a refinement of it) is known as Einstein's hole argument. It is straightforward to include matter - we have a larger set of differential equations but they still have the same form in all coordinates systems, so the same argument applies and again we obtain two solutions with the same initial conditions which impose different spacetime geometries. It is very important to note that we could not have generated these extra distinct solutions if spacetime were fixed and non-dynamic, and so the resolution to the hole argument, background independence, only comes about when we allow spacetime to be dynamic.
Before we can go on to understand this resolution we need to better understand these extra solutions. We can interpret these solutions as follows. For simplicity we first assume there is no matter. Define a metric function 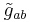 whose value at
whose value at  is given by the value of
is given by the value of 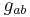 at
at 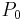 , i.e.
, i.e.
- Eq(3)
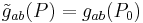 .
.
(see figure 1(a)). Now consider a coordinate system which assigns to  the same coordinate values that
the same coordinate values that 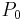 has in the x-coordinates (see figure 1(b)). We then have
has in the x-coordinates (see figure 1(b)). We then have
- Eq(4)

where 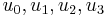 are the coordinate values of
are the coordinate values of 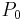 in the x-coordinate system.
in the x-coordinate system.
When we allow the coordinate values to range over all permissible values, Eq(4) is precisely the condition that the two metric functions have the same functional form! We see that the new solution is generated by dragging the original metric function over the spacetime manifold while keeping the coordinate lines "attached", see Fig 1. It is important to realise that we are not performing a coordinate transformation here, this is what's known as an active diffeomorphism (coordinate transformations are called passive diffeomorphisms). It should be easy to see that when we have matter present, simultaneously performing an active diffeomorphism on the gravitational and matter fields generates the new distinct solution.
The resolution to the hole argument (mainly taken from Rovelli's book) is as follows. As GR does not determine the distance between spacetime points, how the gravitational and matter fields are located over spacetime, and so the values they take at spacetime points, can have no physical meaning. What GR does determine, however, are the mutual relations that exist between the gravitational field and the matter fields (i.e. the value the gravitational field takes where the matter field takes such and such value). From these mutual relations we can form a notion of matter being located with respect to the gravitational field and vice-versa, (see Rovelli's for exposition). What Einstein discovered was that physical entities are located with respect to one another only, independent of the spacetime manifold. This independence is background independence.
Since the hole argument is a direct consequence of the general covariance of GR, this led Einstein to state:
"That this requirement of general covariance, which takes away from space and time the last remnant of physical objectivity, is a natural one, ..."[3]
The term "active diffeomorphism" has been used, instead of just "diffeomorphism", to emphasize that this is not a case of simple coordinate transformations. It is active diffeomorphisms which are the gauge transformations of GR and they should not be confused with the freedom of choosing coordinates on the space-time M. Invariance under coordinate transformations is not a special feature of GR as all physical theories are invariant under coordinate transformations. (Indeed, the mathematical definition of a diffeomorphism is a transformation which relates manifolds with equivalent topological and differentiable structure, but not necessarily equivalent metrics. For example, a diffeomorphism can turn a doughnut into a tea cup.)
Whether or not Lorentz invariance is broken in the low-energy limit of loop quantum gravity (LQG), the theory is formally background independent. The equations of LQG are not embedded in, or presuppose, space and time, except for its invariant topology. Instead, they are expected to give rise to space and time at distances which are large compared to the Planck length. At present, it remains unproven that LQG's description of spacetime at the Planck scale has the right continuum limit, described by general relativity with possible quantum corrections.
Manifest background-independence
This is primarily an aesthetic rather than a physical requirement. It is analogous to requiring in differential geometry that equations be written in a form that is independent of the choice of charts and coordinate embeddings. If a background-independent formalism is present, it can lead to simpler and more elegant equations. However there is no physical content in requiring that a theory be manifestly background-independent - for example, the equations of general relativity can be rewritten in local coordinates without affecting the physical implications.
String theory
Although the physics of string theory can in principle be background-independent, perturbative formulations of this theory do not make this independence manifest because they require starting with a particular solution and performing a perturbative expansion about this background. Non-perturbative formulations such as matrix theory and AdS/CFT resolve that issue and are fully background independent.
Background independent quantum gravity theories
String field theory
The classical background-independent approach to string theory is string field theory. Although string field theory has been useful to understand tachyon condensation, most string theorists believe that it will never be useful to understand non-perturbative physics of string theory.
Loop quantum gravity
A very different approach to quantum gravity called loop quantum gravity is background-independent by design. It dispenses with the concept of a background spacetime metric and instead deals with discrete structures called spin foams. The crucial point is that spin foams don't live in spacetime - they are spacetime. Therefore, high-level properties of spacetime, such as dimensionality and topology, are emergent long-distance features of the corresponding spin foam.
One of the major shortcomings of loop quantum gravity is that, as of 2009, it has not been shown that the theory in fact reduces to Einstein's general relativity in the continuum limit. Since GR equations were used initially to construct the theory, many researchers assume that they can be recovered, but this has neither been explicitly proved nor disproved. In addition, incorporating matter into the theory is an ongoing problem.
History of background independent theories
This dichotomy between background dependent and independent theories is sometimes traced back as far as the antagonism between Newton and Leibniz about absolute vs. relational space. Most physicists would claim that the choice of approach is merely philosophical so far as no different falsifiable claims follow, not unlike the question of interpretations of quantum mechanics. However, two philosophers of science, Imre Lakatos and Elie Zahar, have argued that research programs can be driven by metaphysical questions and so adopting the view of background independence may lead to different results. One result development ties in to the J-Kinn postulate.
See also
External links
References
- ^ Gaul, Marcus; Rovelli, Carlo (2000). Loop Quantum Gravity and the Meaning of Diffeomorphism Invariance. "Towards Quantum Gravity". Lect.Notes Phys.. Lecture Notes in Physics 541: 277–324. arXiv:gr-qc/9910079. doi:10.1007/3-540-46634-7_11. ISBN 978-3-540-66910-4.
- ^ Smolin, Lee (2005). "The case for background independence". arXiv:hep-th/0507235 [hep-th].
- ^ Einstein's Equivalence Principle, Einstein's physical space and Observational Validity of the Schwarzschild Solution
- Notes
- L. Smolin, The case for background independence, arXiv:hep-th/0507235
- C. Rovelli et al., Background independence in a nutshell, Class.Quant.Grav. 22 (2005) 2971-2990, arXiv:gr-qc/0408079
- Edward Witten, Quantum Background Independence In String Theory, arXiv:hep-th/9306122
- J. Stachel, The Meaning of General Covariance: The Hole Story, in J. Earman, A. Janis, G. Massey and N. Rescher (eds.), Philosophical Problems of the Internal and External Worlds: Essays on the Philosophy of Adolf Grünbaum, University of Pittsburgh Press 1993, ISBN 0-8229-3738-7, pp. 129–160.
- J. Stachel, Changes in the Concepts of Space and Time Brought About by Relativity , in C. C. Gould and R. S. Cohen (eds.), Artifacts, Representations and Social Practice; Kluwer Academic 1994, ISBN 0-7923-2481-1, pp. 141–162.
- E. Zahar, Einstein's Revolution: A Study in Heuristic, ISBN 0-8126-9066-4